Copyright
- Cards and deal
- Shuffle the 52 cards and place them face down in a stock. Deal four cards face up in a display and turn the top card of the stock face up. This is the target card.
- Object
- Counting Aces and face cards
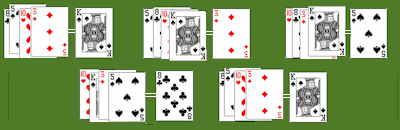
Any one of these five cards can be the solution to an equation
involving the other four. Thus (with K=1): 8+5/10+3=1, (5x8/10)-1=3,
(10+8)/3-1=5,(10/5)+(1x3)=8, 8+5-3x1=10 as 1 each (I dislike games that dispense with face cards), and others at face value, the aim is to use all four display cards in an equation that equals the target card numeral. Only addition, subtraction, multiplication and division are allowed, and any division must be integral (leaving no remainder). Powers, roots, and other mathematical contortions are not allowed as they make the game too easy. Warning: do not attempt to divide by zero, or the world will come to an end. - Play
- Whoever first makes a valid equation wins the target card, discards the display cards face down
to a wastepile, deals another four-card display from the stock, and turns the top card of the stock face up as a new
target.Anyone who makes an invalid equation is barred from further attempts at winning the current target card. If only two play, the defaulter's opponent wins the target.If everyone passes, the target card is replaced at the bottom of the stock and the next top card is turned in its stead. When only two cards remain in stock, add them to the wastepile, shuffle it, and turn it over to form a new stock. Continue until 48 cards have been won and only four remain in stock. These last four are won by whoever made the last equation.If only two play, stop when either player has won 12 cards, or any other agreed number.
- Score
- You each score the number of cards you have won, or, if previously agreed, their total face value.
- Game
- Keep playing till you get tired of it.
- Variant 1
- Counting face cards as 1 each makes life too easy. For more of a challenge, count Jack 11, Queen 12, King 13. The trouble with this is that if they are all too high the task can get impossible. How, for example, do you make 12 from 13.13.13.13? One way is to omit one card and make an equation of the other three - thus 13-(13/13)=12. Another is to accept powers. This often enables you to generate 1, and since 1 raised to any power remains 1 you can have, for example, 13‑(13/13)^13)=12. Also useful is the fact that anything raised to the power of zero is equal to 1, thus allowing you the alternative 13-(13^(13-13))=12.
- Variant 2
- Perhaps a better approach is
to count face cards at their suit value, for which purpose I favour
spades 1, hearts 2, clubs 3, diamonds 4. - Variant 3
- This substantially similar game is called Ascent. Take one card of each suit and arrange all four in a square.
The first player endeavours to form them into an equation equal to 1, counting numerals at face value from Ace=1 to Ten=10 and face
cards at their suit value of spades=1, hearts=2, clubs=3, diamonds=4.If unable, the second player turns the next card of stock, places it on the display card of the same suit, and similarly tries to form an equation equalling 1. If also unable, the first player turns another card, places it on its suit pile, and tries again. This continues until either player makes an equation equalling 1, whereupon the next in turn turns the next card form stock, places it on its suit pile, and seeks to make an equation equalling 2. Play continues in this way until every number has been made up to an agreed total, say 20.A player who succeeds in making up the required target number after the other has (or both have) failed to do so scores the amount of that target.In a sample game consisting of 16 turns one player scored 4+14+16+17=51 to the other’s 6+7+15+18=46. There were two occasion on which neither player succeed at the first attempt, first when the target was 14, next when it was 17.
- Footnote
- If you like equation games, see also Equator, One Up, and Prime Time